Mauchly spent about five days in Iowa. The day after he arrived, Atanasoff took him to his laboratory to see his calculator, which was still under construction. About as big as a large desk, the machine was covered with a sheet. Atanasoff removed the cover, pointed out the various parts of the gadget, and explained what they did. Although Mauchly spent only a short time in the lab that day – it was a Sunday – he saw the device several times that week, either in the company of Atanasoff or his assistant, Clifford Berry, a talented graduate student in electrical engineering. Mauchly and Atanasoff spent most of their time talking about electronic computing. Atanasoff also showed Mauchly a paper he had written the previous summer about the machine, an extraordinary document that was practically a step-by-step blueprint for the construction of an electronic calculator.
The paper, entitled “Computing Machine for the Solution of Large Systems of Linear Algebraic Equations,” was really a grant proposal. Beginning with a discussion of the calculator’s many applications, it contains an exhaustive description of the device, right down to the wiring diagrams. In brief, Atanasoff was trying to build a digital electronic calculator founded on binary math and Boolean algebra, using arithmetic units made of vacuum tubes; a memory composed of condensers (now called capacitors, which can store electrical charges); and an input and output system based on punch cards. Atanasoff’s goal was a machine that could solve up to twenty-nine simultaneous linear equations at a time – which would have made it quite useful. Moreover, Atanasoff had come to realize that, with some modification, his invention could even be used to solve differential equations and, therefore, calculate firing tables, an idea that Mauchly found particularly intriguing.

Like Mauchly, Atanasoff inherited his passion for science from his father, an electrical engineer who had emigrated to the United States from Bulgaria in the late 1800s. The oldest of ten children, Atanasoff grew up in Florida, where his father worked for a phosphate mine near Lakeland. In 1913, when Atanasoff was nine, his father loaned him his slide rule and gave him a few tips on how to use it. For the most part, however, Atanasoff was left to his own devices. Guided by an instruction booklet that came with the rule and a college algebra text that belonged to his father, he learned how to use the rule to perform fairly complicated mathematical operations, including the calculation of logarithms. That rule was the beginning of his lifelong interest in artificial computation.
By the time he went to college, Atanasoff had decided to become a theoretical physicist. But the physics program at the University of Florida in Gainesville wasn’t particularly good at the time, so he switched to the next best thing on the curriculum, electrical engineering. After Gainesville, he took a master’s in physics from Iowa State and a doctorate from the University of Wisconsin at Madison. His thesis was on the electrical properties of helium (which isn’t an electrical conductor but can, under certain circumstances, sustain an electrical field); his work was intensively mathematical, and he spent weeks toiling over equations, with only a desk calculator for help. Iowa State offered him an assistant professorship in math and physics and, at twenty-seven, Atanasoff became what he had always wanted to be, a theoretical physicist.
His interest in electronic calculation developed slowly. Over the years, Atanasoff experimented with various mechanical and theoretical methods of solving complex mathematical problems. The statistics department at Iowa State had an IBM tabulator and other calculating equipment, and Atanasoff and a colleague managed to get the machines to solve a problem in spectral analysis (Mauchly’s line of work). In the course of his teaching and research, Atanasoff also did a lot of work in differential equations, solutions for which could be obtained, in a roundabout way, with linear algebraic equations. This common bit of mathematical knowledge led Atanasoff, who knew a great deal about electronics, to ponder the possibility of building an electronic calculator that could solve these equations.
By 1937, he had a good idea of the kind of machine he wanted to build, and he fleshed out the details over the next two years. He had an engineer’s instinct for the heart of the matter that was almost as good (or as good) as Zuse’s and their overall technical approach was quite similar. In early 1939, therefore, Atanasoff was ready to proceed. On the basis of a preliminary proposal, he obtained a $650 grant from Iowa State in the summer. He hired Berry in August or September, and they managed to finish a small prototype one or two months later, a crude device that could add and subtract sixteen-digit binary numbers (the equivalents of eight-digit decimal figures). It operated well and, although it was only a test-bed, was the first machine to calculate with vacuum tubes.
Pleased with his work and certain of success, Atanasoff wrote a detailed grant proposal (the one he showed Mauchly) in the summer of 1940, requesting another $5,000. (He had received about $1,500 from Iowa State and other sources so far.) And it was at this point in the project, while the machine was half completed and Atanasoff was trying to raise more money, that Mauchly visited Ames. The money came through several months later, and Atanasoff and Berry completed the machine in the spring of 1942. At the time, Atanasoff called his invention simply a computing machine, but, years later, he decided to give it a catchier name – the ABC, for Atanasoff-Berry Computer.
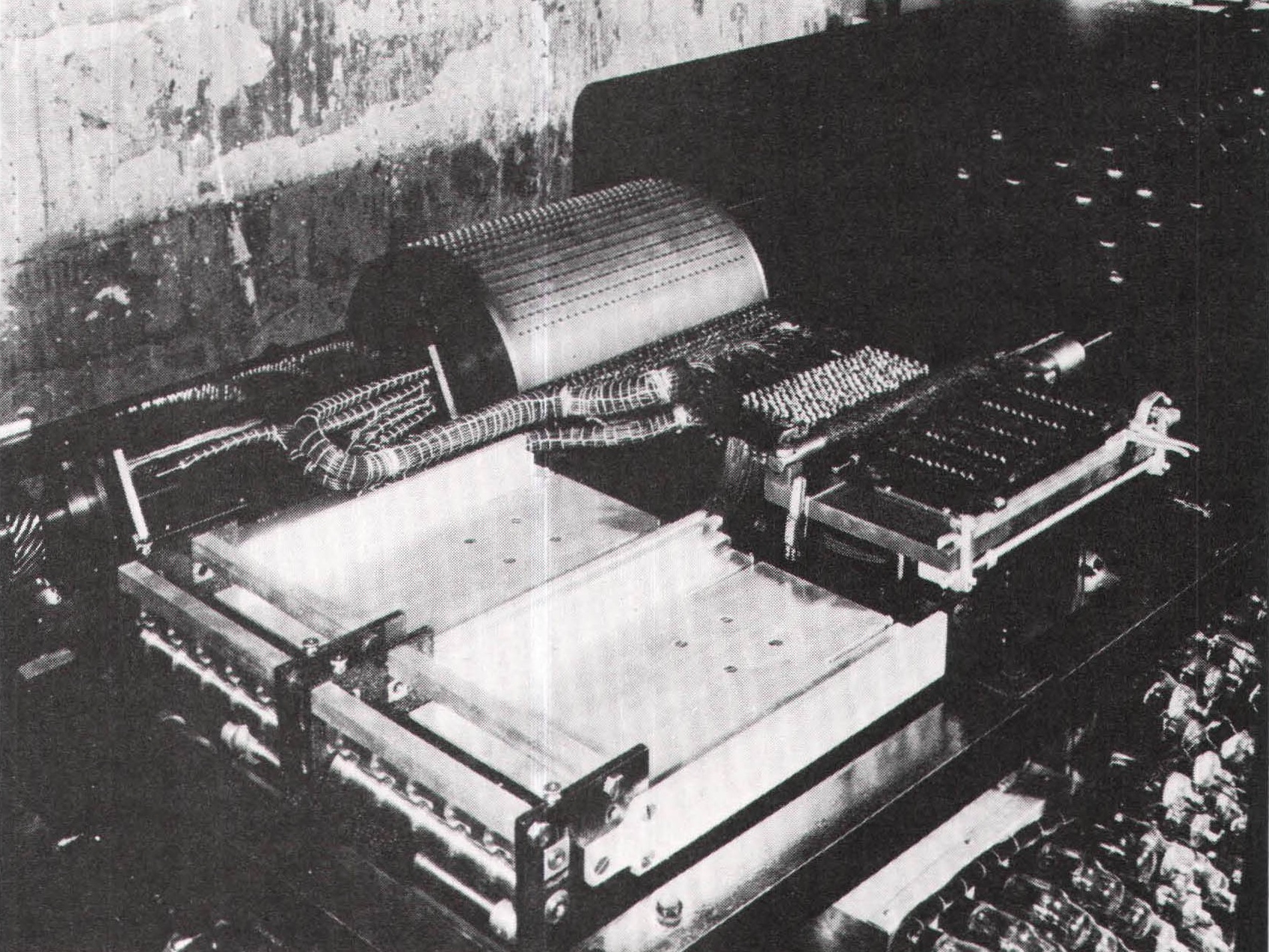
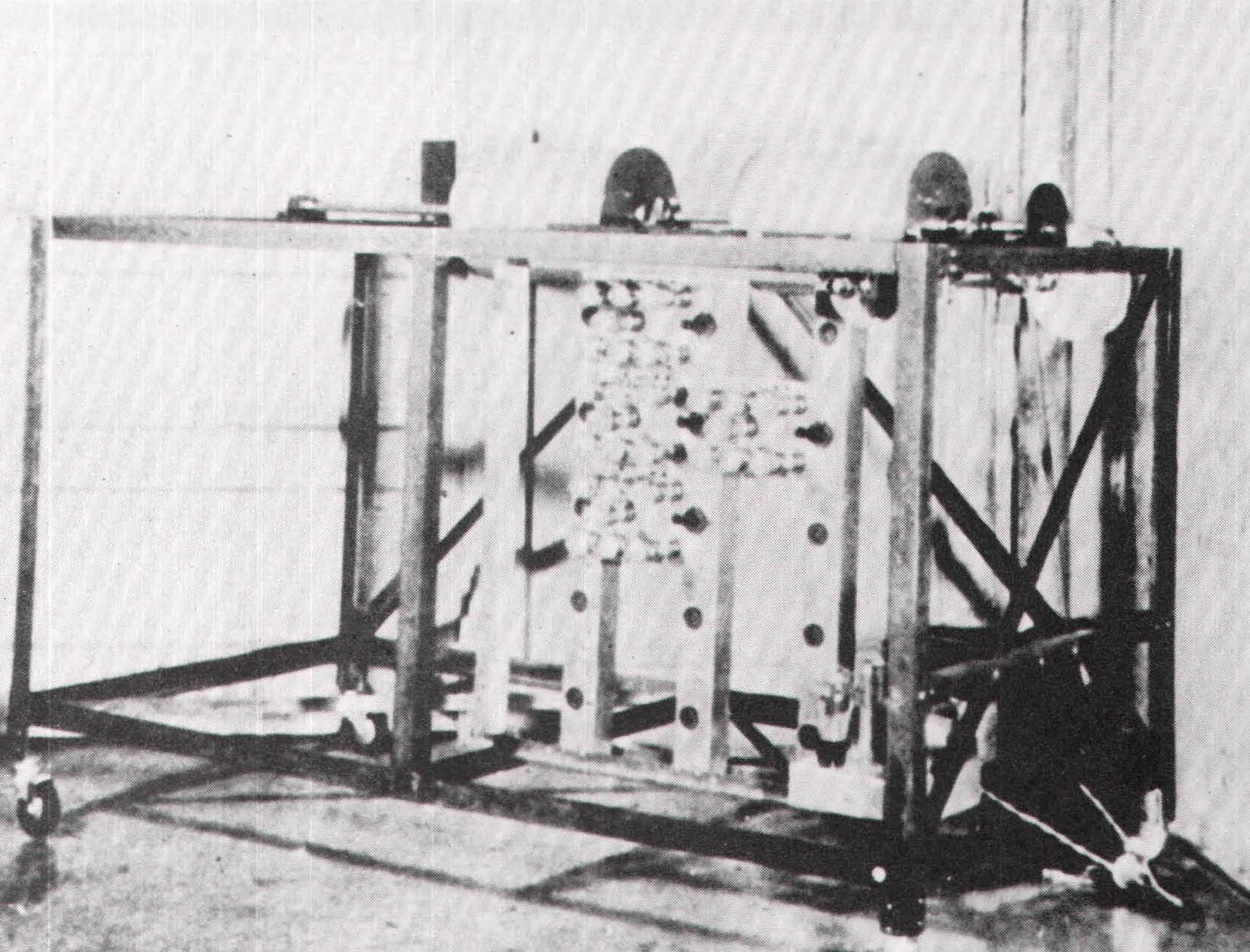
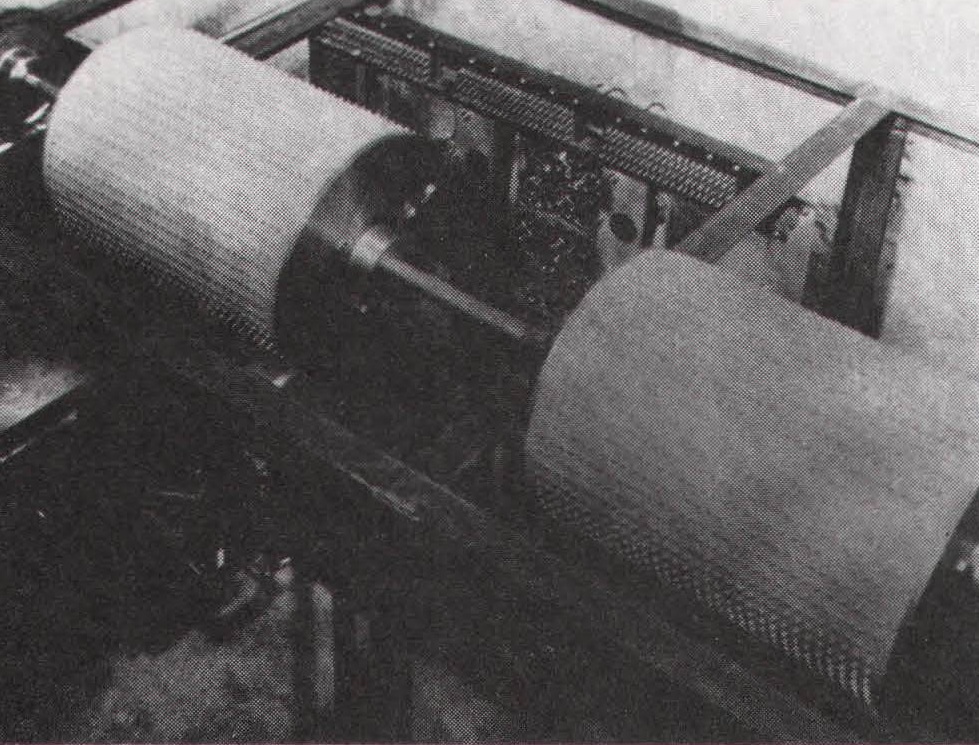
The ABC was about the size of a large desk. All of its parts were mounted on a metal frame. The operator’s control console, which contained numerous switches, buttons, meters, and lights, was on top. The addition and subtraction unit, which contained two hundred and ten tubes, lay under the console, with another bank of thirty tubes, which controlled the card reader and puncher, occupying the space next to the addition and subtraction unit. There was a punch card reader and puncher next to the console, within easy reach of the operator, and, behind the card units, the most interesting part of the ABC – two rotating drums that served as the memory. These drums were primitive versions of the rotating drum memories that appeared in the computers of the late 1940s and early 1950s. About twelve inches long and six inches in diameter, each drum could store thirty fifty-bit numbers (the equivalent of a fifteen-digit decimal number) in condensers set into their skins. Another thirty tubes helped maintain the charges stored in the condensers, which tended to drain away.
Except for the binary-card puncher, a rather complicated piece of equipment that created holes electrically rather than mechanically, burning them in, everything worked perfectly. The card puncher failed about once every hundred thousand times, which may not seem particularly serious – after all, it worked more than 99 percent of the time – but it prevented the ABC from solving large sets of linear equations. These equations used a veritable ocean of binary numbers, and any malfunction in the puncher meant that the numbers entering and exiting the ABC were sometimes wrong. Atanasoff and Berry tried to exorcise the puncher’s bugs, experimenting, for example, with cards made of different materials; but they failed, and the machine could never solve more than a few simultaneous equations. Nevertheless, Atanasoff and Berry had built a working special-purpose digital electronic calculator, and their achievement was historic.
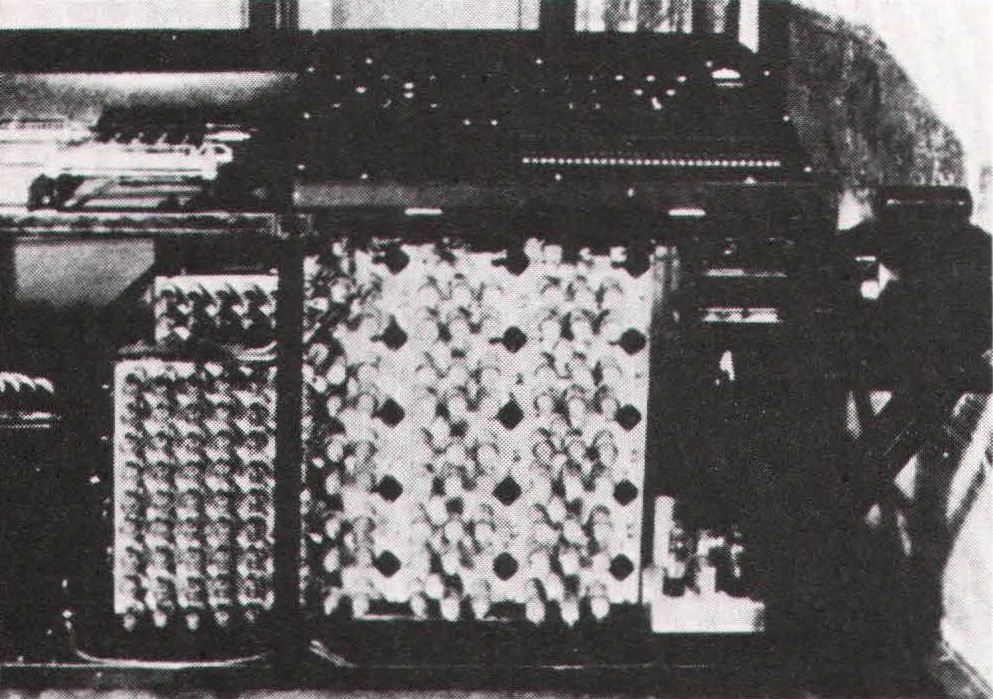
The ABC had several other shortcomings, the foremost being its lack of programmability and automaticity. Although it could carry out some operations on its own, it was controlled almost every step of the way by the operator, who stood at the console, pressing buttons, turning knobs, watching meters, and inserting and removing the punch cards. Like a car, the ABC needed a driver. In this respect, Zuse’s machines were much more sophisticated – they were programmable and automatic. The ABC also lacked a central processor, the machine’s arithmetic tasks being handled chiefly by thirty independent sets of tubes in the addition and subtraction units. Although this arrangement limited the ABC’s flexibility, it wasn’t necessarily a major drawback, because the machine was designed to solve only linear equations.
Finally, the ABC was painfully slow. Constrained by limited financial and technical resources, Atanasoff took a conservative approach to the ABC’s design, combining a high-speed electronic arithmetic unit with a much slower, and quite inexpensive, set of memory drums and card readers and punchers. As a result, he couldn’t capitalize on the potential speeds of a fully electronic machine – speeds that would have catapulted his invention into a transcendent technological realm – and he was forced to set the ABC’s internal clock, the timer that regulated its operations, at a mere sixty pulses a second, which enabled the ABC to add two fifty-bit numbers a second. By contrast, ENIAC ran at a speed of 100,000 beats a second and could add 5,000 ten-digit decimal numbers, each containing about twenty bits, in the same amount of time.