
The third great calculator inventor of the seventeenth century was Gottfried Wilhelm von Leibniz. The range and richness of his intellect was nothing less than phenomenal. Leibniz was a master of almost a dozen disciplines: logic, mathematics, mechanics, geology, law, theology, philosophy, history, genealogy, and linguistics. His greatest achievement was the invention of differential calculus, which he created about twenty years later than Newton but in a much more practical form. Indeed, the stubborn refusal of English mathematicians to adopt Leibniz’s notation retarded the development of mathematics in England for more than a hundred years. Leibniz was driven by a monumental obsession to create, to build, to analyze, to systematize – and to outdo the French. A bibliography of his writings would go on for pages; many of his manuscripts have still not been published and his letters may be measured by the pound.
Born in 1646, two years before the end of the Thirty Years’ War, Leibniz was the son of a notary (a minor judge) and professor of moral philosophy at the University of Leipzig. His father died when he was six and he was raised by his mother, a pious Lutheran who passed away when he was eighteen. Like Pascal, he was a prodigy, and his mother gave him the run of his dead father’s library – not an easy decision in those days, when children were brought up on a very tight leash and their reading restricted to approved books, lest their minds be contaminated by impure thoughts (of which Leibniz undoubtedly had many). He had a natural aptitude for languages and taught himself Latin when he was eight and Greek a few years later. At thirteen, he discovered one of his lifelong passions, the study of logic. He was, as he later wrote, “greatly excited by the division and order of thoughts which I perceived therein. I took the greatest pleasure in the predicaments which came before me as a muster-roll of all the things in the world, and I turned to ‘Logics’ of all sorts to find the best and most detailed form of this list.”
He entered the University of Leipzig when he was fifteen, majoring in law. He was by nature a weaver of grand systems, and in 1666 he wrote a treatise, De Arte Combinatoria (On the Art of Combination) offering a system for reducing all reasoning to an ordered combination of elements, such as numbers, sounds, or colors. That treatise is considered one of the theoretical ancestors of modern logic, a primitive form of the logical rules that govern the internal operation of computers. That same year, all his requirements for the doctorate in law having been completed, Leibniz proudly presented himself for the degree. He was only nineteen, and the elders in charge of the gates of the bar turned him down on account of his age. Furious, he went to the University of Altdorf, in Nurnberg, where his dissertation (De Casibus Perplexis, or On Perplexing Cases) immediately won him a doctorate and an offer of a professorship.
However, Leibniz disliked the stuffiness and pettiness of academia and sought a diplomatic career. One of the most important diplomats of the time, Johann Christian von Boyneburg, took him under his wing and secured a post for him at the court of the archbishop of Mainz, the Prince Elector Johann Philipp von Schonborn. (The electors chose the Holy Roman Emperor, who ruled over the states encompassing Germany and most of Central Europe.) Leibniz was put to work codifying and revising the laws of Nurnberg – hardly a reforming effort, since the many codifications of the period were designed to solidify the power of the ruling classes. For the rest of his life, the broad-shouldered, bandylegged Leibniz served in one or another capacity as an official in the courts of the German princes, a genius in the service of mediocrities.
France was the greatest power in seventeenth-century Europe, and the Holy Roman Empire feared that she would invade Holland and, possibly, Germany. Hoping to distract Louis XIV, Leibniz and the archbishop’s advisors tried to interest him in a military campaign in the Mideast. In terms full of religious emotionalism, they recommended that France launch a holy crusade against Egypt and Turkey. In 1672, the archbishop dispatched Leibniz on a solitary mission to Paris to discuss the plan with the king. Not surprisingly, the trip was an utter failure; Louis XIV didn’t even bother to acknowledge the young German’s arrival, let alone grant him an audience. But Paris proved to be a muse of the highest order, and it was there, between 1672 and 1674, that Leibniz built his first calculator (or, rather, had a craftsman build it for him).
He explained the genesis of the Stepped Reckoner, as he called his invention, in a note written in 1685:
When, several years ago, I saw for the first time an instrument which, when carried, automatically records the numbers of steps taken by a pedestrian [he’s referring to a pedometer, of course], it occurred to me at once that the entire arithmetic could be subjected to a similar kind of machinery so that not only counting but also addition and subtraction, multiplication and division could be accomplished by a suitably arranged machine easily, promptly, and with sure results. The calculating box of Pascal was not known to me at that time. I believe it has not gained sufficient publicity. When I noticed, however, the mere name of a calculating machine in the preface of his”postumous thoughts” [the Pensees]… I immediately inquired about it in a letter to a Parisian friend. When I learned from him that such a machine exists I requested the most distinguished Carcavius by letter to give me an explanation of the work which it is capable of performing. He replied that addition and subtraction are accomplished by it directly, the other [operations] in a round-about way by repeating additions and subtractions and performing still another calculation. I wrote back that I venture to promise something more, namely, that multiplication could be performed by the machine as well as addition, and with greatest speed and accuracy.

Conceptually, the Stepped Reckoner was a remarkable machine whose operating principles eventually led to the development of the first successful mechanical calculator. The key to the device was a special gear, devised by Leibniz and now known as the Leibniz wheel, that acted as a mechanical multiplier. The gear was really a metal cylinder with nine horizontal rows of teeth; the first row ran one-tenth the length of the cylinder, the second two tenths, the third three-tenths, and so on until the nine-tenths length of the ninth row. The Reckoner had eight of these stepped wheels, all linked to a central shaft, and a single turn of the shaft rotated all the cylinders, which in turn rotated the wheels that displayed the answers.
Say you wanted to multiply 1,984 by 5. First, you entered the multiplicand (1,984) through the numbered dials, or pointers, on the top face of the machine. Then you put a metal peg in the fifth hole of the large dial on the far right; the peg served as a built-in reminder that the multiplier was 5 and prevented you from entering a larger figure. You next took hold of the wooden handle on the big dial on the front – this was the multiplier dial, which was linked to the central shaft – and turned it once. The answer appeared in the little windows behind the numbered pointers. If the multiplier contained more than one digit – say, 555 – you had to shift the Reckoner’s movable carriage one place to the left for every decimal place, and turn the multiplier handle once for every digit. (Along with the stepped cylinder, the movable carriage ended up in many other calculators, not to mention the typewriter.)
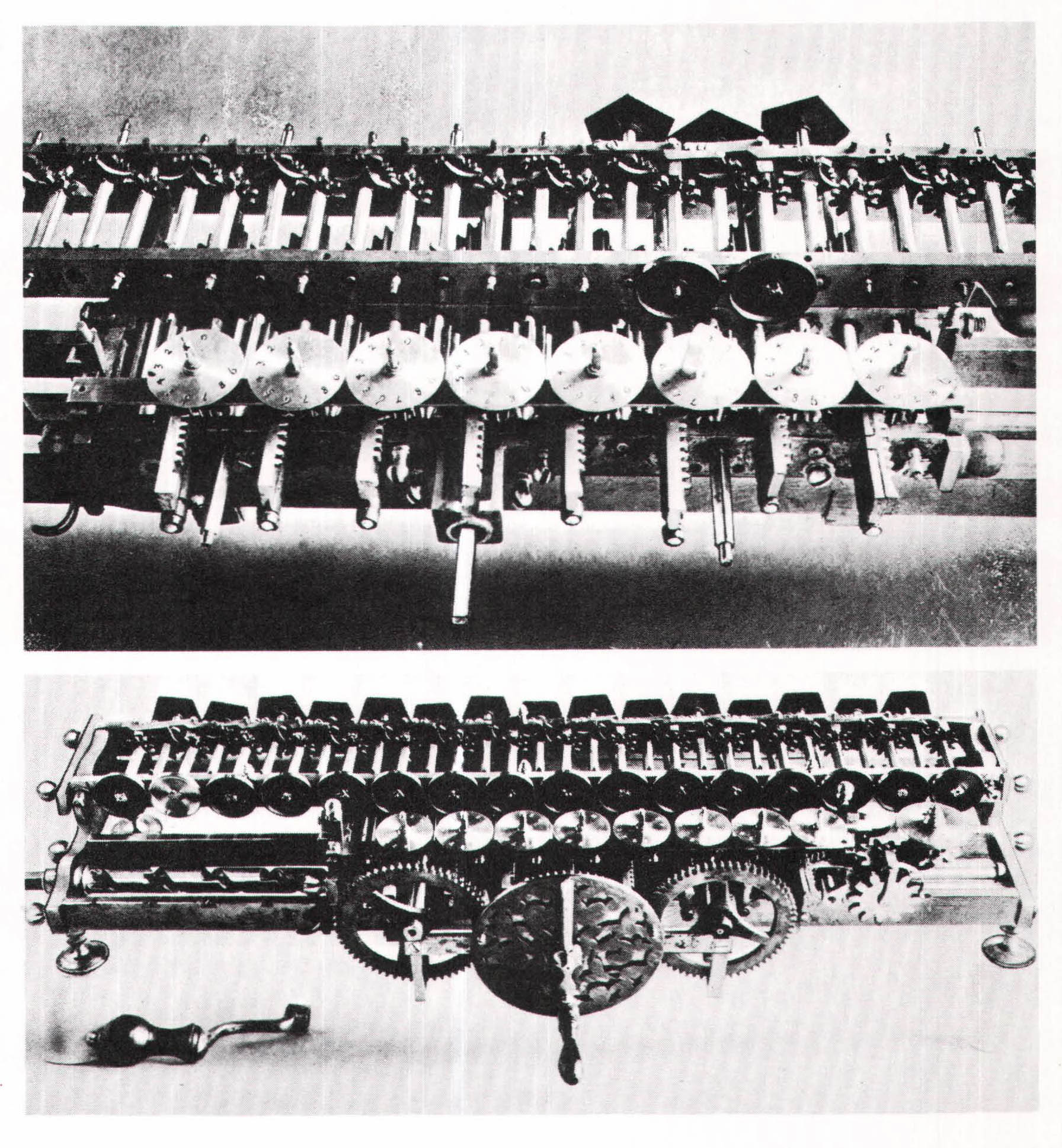
Although the Reckoner could process fairly large numbers – multipliers of four or five digits, multiplicands of up to eleven or twelve digits – it wasn’t fully automatic, and you had to fiddle with a row of pentagonal widgets at the back of the machine to help it carry and borrow digits. Nevertheless, it was far more sophisticated than the Calculating Clock or the Pascaline, capable of all four arithmetic operations and much closer to what we would consider to be a calculator. But the Reckoner suffered from one great drawback, much more serious than its inability to carry or borrow numbers automatically – it didn’t work. Leibniz’s ambition outran his engineering skill, and the only surviving version of the calculator, on display at a museum in Hannover, West Germany, is an inoperative relic.
In 1764, forty-eight years after Leibniz’s death, a Reckoner was turned over to a clockmaker in Gottingen for overhauling. The job wasn’t done, and Leibniz’s pride and joy wound up in the attic of the University of G6ttingen, where a leaky roof led to its rediscovery in 1879. Fourteen years later, the university gave the machine to the Arthur Burkhardt Company, the country’s leading calculator manufacturer, for repair and analysis. Burkhardt reported that, while the gadget worked in general, it failed to carry tens when the multiplier was a two- or three-digit number. The carrying mechanism had been improperly designed. It’s unknown whether Leibniz, who worked on the Reckoner off and on for twenty years, built more than one calculator – one that was flawed and one (or more) that worked. In all likelihood, given the high costs of fashioning a device as complicated as the Reckoner, Leibniz made only one and never managed to perfect it.