When we last examined the state of mechanical calculation, Thomas de Colmar had invented and marketed a reliable four function calculator, called the Arithmometer. Based on the Leibniz wheel, it was the first major advance in calculator technology since the late 1700s and Thomas’s design was widely emulated. For decades, Thomas-type machines were the only truly useful calculators on the market. But the situation changed dramatically after 1875. As a result of advances in machine tooling and mechanical engineering, it became possible to do more with gears and axles than ever before. A veritable explosion in calculator design and manufacturing took place, as a growing number of inventors sensed a need and sought to fill it.

Once again, the major breakthroughs occurred in America. In 1872, Frank Stephen Baldwin, of St. Louis, conceived of a new kind of calculator mechanism, the pinwheel, which operated like the Leibniz wheel. When the operator entered a number -let’s say, five – via a lever on the face of the machine, five springloaded pins, or sprockets, protruded from the edge of a wheel inside the device. The operator then turned a crank and the pinwheel rotated the relevant inner gears five notches. Baldwin’s clever machine was the first major innovation since the Arithmometer, and it inspired a host of imitators. In 1875, he obtained a patent and set up a small factory in Philadelphia, inaugurating the American calculator industry. However, he failed to make a go at it until 1912, when he and Jay Randolph Monroe, an auditor for the Western Electric Company, established the Monroe Calculating Machine Company. By this time, poor Baldwin was seventy- four years old.
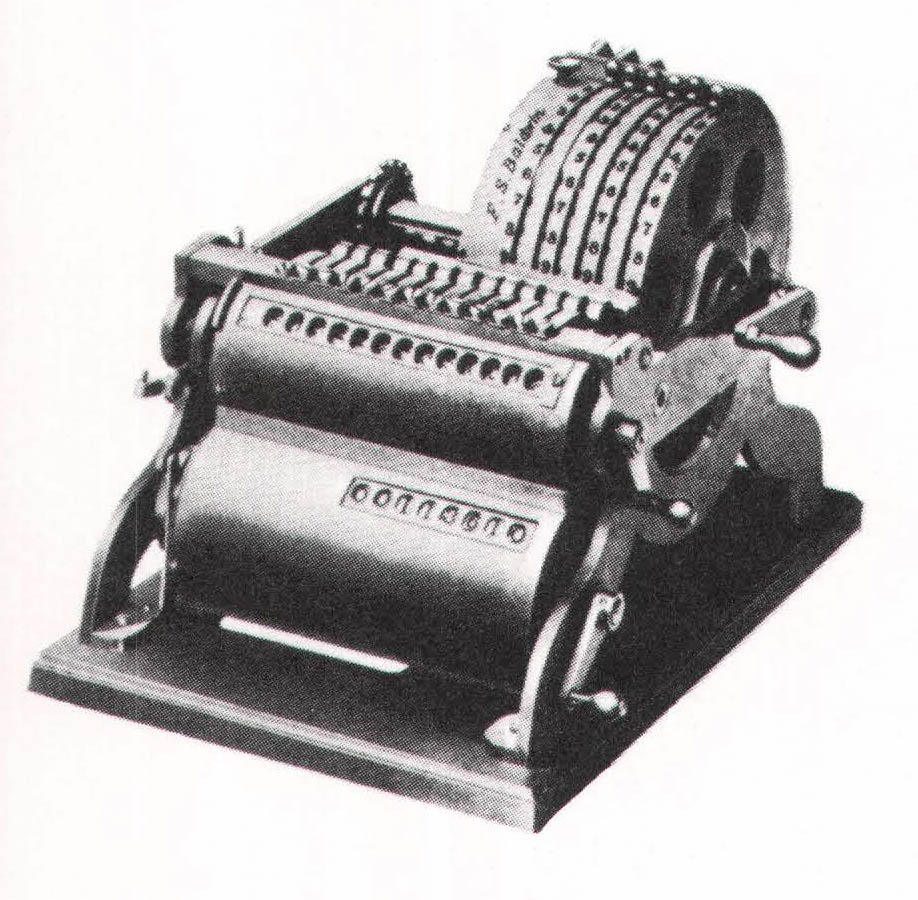
Incidentally, in 1878 a Swedish engineer named Willgodt Theophil Odhner developed a calculator very much like Baldwin’s. Odhner had greater financial success than Baldwin and several European manufacturers produced his machines. Although both men have been credited with the invention of the pinwheel principle, the honor rightly belongs to Baldwin; in his first U.S. patent, Odhner didn’t lay claim to the pinwheel idea.
At about the same time Baldwin came up with the pinwheel, other inventors managed to design calculators with the mechanical equivalent of built-in multiplication tables. The first of these machines, which greatly speeded up multiplication and division, was invented in 1878 by Ramon Verea, a Spaniard living in New York City. His device “looked up” the product of two numbers on a pair of cylinders, and multiplied the inner gears accordingly. An interesting fellow, Verea had no commercial ambitions; he told a New York Herald reporter that he “did not make the machine to either sell its patent or put it into use, but simply to show that it was possible and that a Spaniard can invent as well as an American.” Touché!
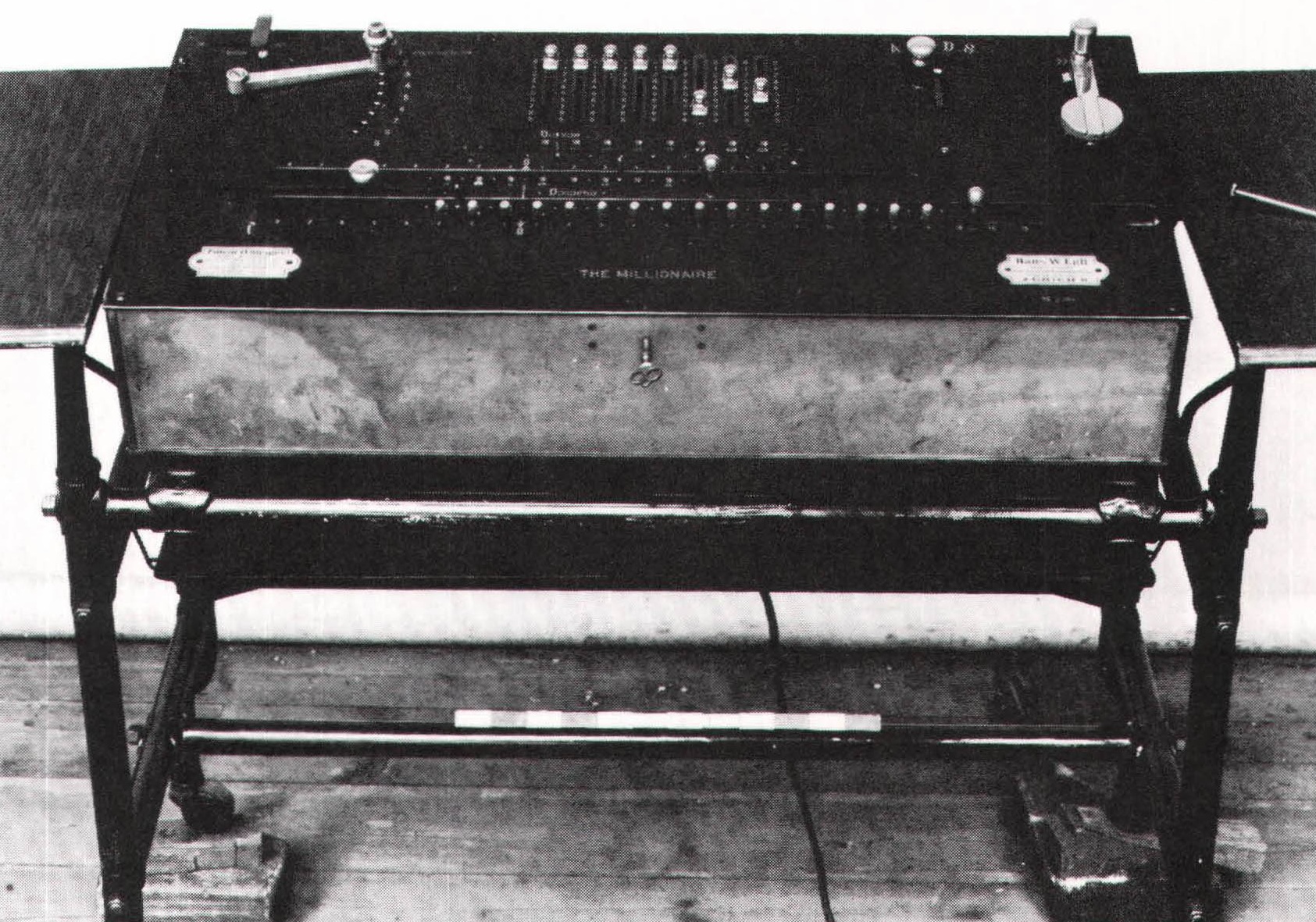
And the French were no less clever. In 1889, Leon Bollee, an eighteen-year-old mechanical genius, also invented a calculator with an internal multiplication table. His machine captured a gold medal at the 1889 Paris Exhibition, but Bollee, an energetic and restless man, soon went on to other things – such as constructing race cars and establishing the racetrack at Le Mans. It was left to Otto Steiger, a Swiss engineer, to design and market the first practical multiplication-table calculator. Known as The Millionaire, it was introduced in 1893 and found a welcome home in accounting rooms and universities all over the world. The Millionaire had an unusually long life for a machine: 4,655 were sold before it was taken out of production in 1935.
All of these machines operated on different principles, testimony both to the ingenuity of their inventors and to the anarchy of the state of the art of mechanical calculation. Although Baldwin’s and Steiger’s calculators could perform all four arithmetic functions, they failed to crack the mass market, and the reason was chiefly technical. Along with all their rivals, these calculators had at least two major limitations. First, they lacked a truly convenient method for entering numbers, which made them somewhat awkward to use; second, they lacked a printer for recording results, which meant that you had to keep track of your results and write the answers down on a sheet of paper. There were too many opportunities for error.

In 1885, Dorr E. Felt, a veritable fountain of creativity who worked as a mechanic for the Pullman Company, in Chicago, made the pivotal breakthrough with the invention of a key-driven calculator. Felt’s Comptometer was the epitome of convenience: all you had to do was tap in the numbers on a typewriterlike keyboard and the gadget did the rest. The mere act of pressing the keys, which were linked to springs, drove the device. Four years later, Felt solved the second drawback with a built-in printer that automatically recorded the entries and answers. He teamed up with Robert Tarrant, a Chicago businessman, and the Felt & Tarrant Manufacturing Co. started production in 1889. By 1930, when Felt died, the firm had $3.1 million in sales and 850 employees.


Without direct competition, Felt & Tarrant might have dominated the market, but the idea of a calculator with a numeric keyboard and a built-in printer also occurred to William S. Burroughs (1857-98). Burroughs, a clerk in a bank in upstate New York, knew from firsthand experience the inadequacies of the available calculators. When he was twenty-six, he moved to St. Louis and worked briefly in his father’s model-making and casting shop, where he met many inventors, including Baldwin. Suitably inspired, Burroughs began tinkering with a calculator of his own. In 1884, he developed a version with a keyboard driven by a handle (not by springs, as in Felt’s model, which was not yet on the market). With the substantial backing of three St. Louis merchants, Burroughs rushed his machine into production in 1887.
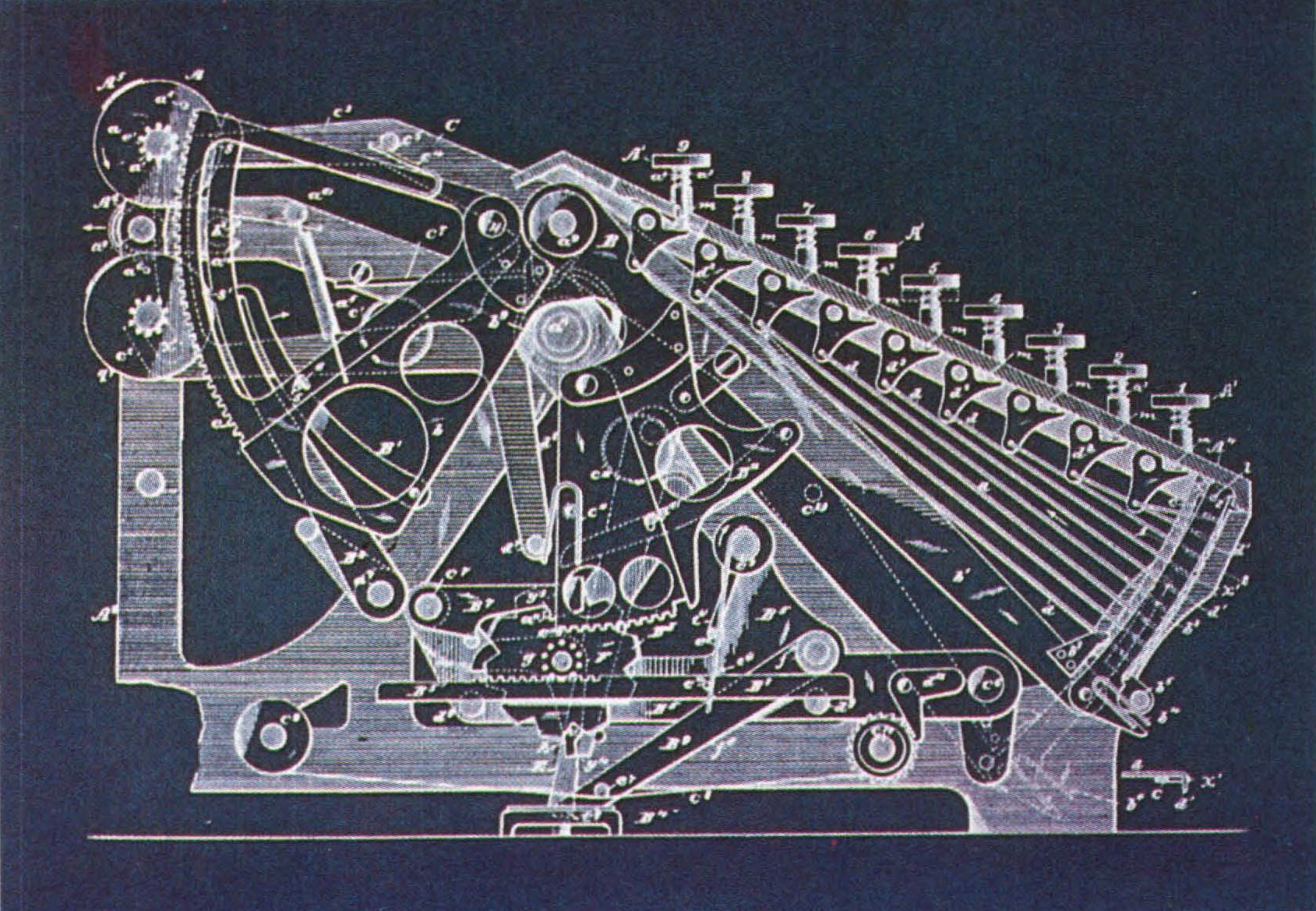
But his haste proved to be an expensive mistake; the machines didn’t stand up to everyday use and had to be scrapped. Furious, Burroughs walked into the stockroom one day and tossed his machines out of the window, one by one. In 1892, he patented another keyboard calculator, this time with a built-in printer, and this model turned out to be a winner. It far outsold every other calculator on the market; about 2,000 were purchased in 1901, 3,000 in 1902, 4,500 in 1903. Unfortunately, Burroughs, who suffered from poor health, did not live long enough to enjoy his success; he died in 1898 at the age of forty-one. By 1913, the Burroughs Adding Machine Company, which had moved to Detroit, had some 2,500 employees and $8 million in sales – it was as big as all of its competitors combined.

Burroughs, Monroe, Felt and Tarrant, and the other calculator manufacturers found a ready market for their wares in banks, companies, accounting departments, and universities. By the 1920s, electric calculators were available; you just pushed some buttons and the machines did most of the work, printing out the results on neat rolls of paper. Although anyone with a couple of hundred dollars to spare could buy a machine that did basic arithmetic, these calculators weren’t much good at more complicated mathematical problems. Some scientists and engineers, thinking they had the seeds of a solution, ganged conventional calculators together in cascading rows; but such Rube Goldbergian contraptions were expensive and cumbersome. The computer demanded a completely different approach, from the composition of its innards to the nature of its number system.