Napier’s work had many practical offshoots. In 1620, three years after Napier’s death, the English mathematician William Gunter (1581-1626) developed a physical analog of logarithms. Gunter, a colleague of Briggs’s at Gresham College, drew a grid of lines on a sheet of parchment and multiplied and divided numbers by adding and subtracting lengths with a compass. As with logs, the operative principle is the exponent, and each point on Gunter’s scale, or line, is exponentially distant from the others. The “Gunter” became a popular navigator’s tool. Two years later, another English mathematician, William Oughtred (1574-1660), rearranged Gunter’s lines into a pair of circles, refigured their numbers, and came up with a device that found a warm spot in the hearts of scientists and engineers for hundreds of years – the slide rule, which enables you to perform rough but rapid multiplication and division by sliding a numbered stock between two fixed slats.

Oughtred was one of those brilliant country clergymen who dabbled in mathematics. A deeply religious man, he wavered between a career in academia and the church; but he decided to follow his heart and, after serving as a fellow for several years at Cambridge, his alma mater, joined the ministry. He wound up as rector in Albury, Surrey, where he continued his research and corresponded with mathematicians all over Europe. He gained a considerable reputation as a mathematician and attracted many students. (Albury, which lies just south of London, wasn’t far from Oxford, Cambridge, and the other intellectual centers of England.) There, Oughtred tutored the sons of the local nobility and taught promising young mathematicians for free.
One of his more imaginative students was a fellow named Richard Delamain, who went on to become a mathematics teacher in London and who, in 1630, published a paper describing a circular slide rule. Oughtred, claiming to have invented the circular rule eight years earlier, accused Delamain of stealing his idea. The two men and their supporters fought it out for years, in print and in person; one of Oughtred’s wittier defenders described Delamain as “the pickpurse of another man’s wit.” Oughtred finally freed himself from his shyness for the printed word and issued a paper on the circular rule in 1632 and another on a rectilinear version in 1633. Meanwhile, Delamain, thanks to his newfound fame as the creator of the circular rule, was appointed quartermaster general and mathematics tutor to King Charles.
It appears that Delamain invented the circular rule later than, but independently of, his teacher, who is the undisputed creator of the more useful and popular rectilinear version. In any event, the first rectilinear rule consisted of two wooden scales, marked with logarithmic lines, that were held in the user’s hands and slid
back and forth against each other; in 1654, the rectilinear rule as we know it today – a sliding stock between two fixed slats – appeared. As time went by, both types of rule were modified and improved, and various mathematical scales, in addition to the original ones for multiplication and division, were included. Many special-purpose rules were also developed, in various shapes and sizes, for the use of scientists and engineers.
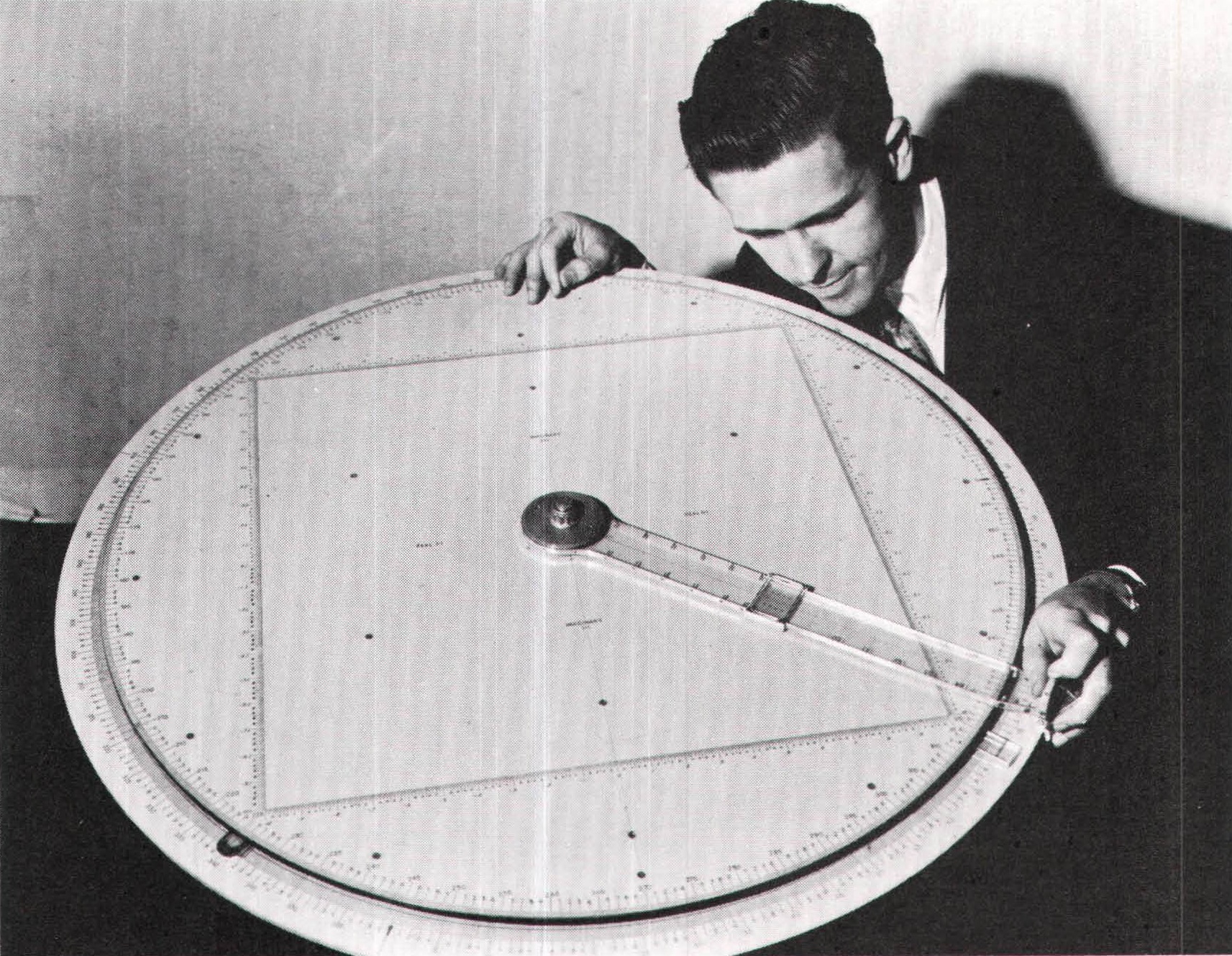
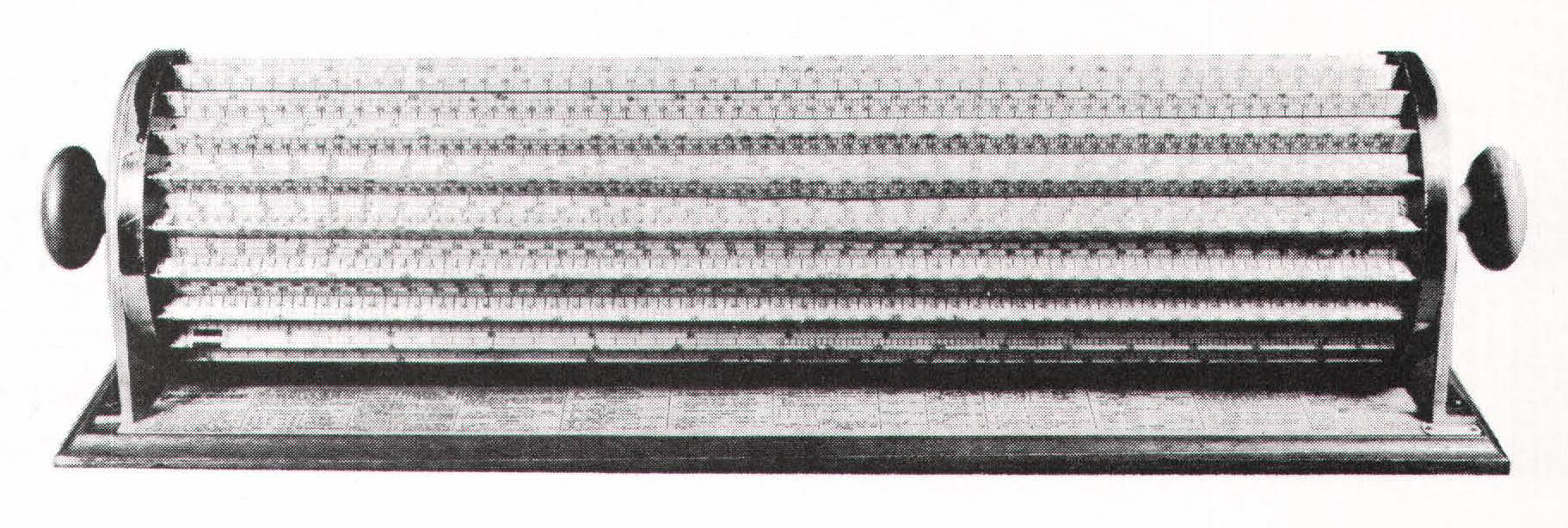
With their numerical scales engraved or printed on wood or ebony, slide rules were accurate only for computations to the second or third decimal place. (Plastic slide rules came along in the 1950s.) However, because many practical problems in science and engineering don’t require exact answers, the thumbnail computational ability of slide rules wasn’t necessarily a drawback, and the slide rule enjoyed a long and fruitful life. By the late nineteenth century, the need for faster and more accurate figuring led to ever bigger and more complicated rules and, by the middle of the twentieth century, the device reached preposterous dimensions. In 1952, for instance, an engineer at the Northrop Aircraft Company, of Hawthorne, California, created a circular rule that was about the size of a tabletop. It was quite possibly the largest rule ever made.
Incidentally, the invention of the slide rule wasn’t Oughtred’s only contribution to mathematics. In the early seventeenth century, there was little consensus on the kind of notation to use for even the most basic arithmetic operations, and Oughtred is credited with introducing the times sign (x) for multiplication and the double colon (::) for ex pressing ratios, a symbol now rarely used. Napier also did his part to standardize numerical notation, giving us a simple and unambiguous way to write decimals – the decimal point.