In the spring of 1935, Zuse graduated from the Technical College and went to work for the Henschel Aircraft Company, in Berlin, as a stress analyst. He spent most of his time composing and solving linear equations. Enough was enough, and he started building his first calculator in 1936, when he was twenty-six years old. (If linear equations were used for the analysis of static structures, why was Zuse fiddling with them in an aircraft plant? At certain high speeds, aircraft wings will flap in the wind like flags in a breeze. By considering wings as static structures and pinpointing their “resonant frequency” with linear equations, engineers can design wings that will be stable enough for any wind speeds a plane is likely to encounter.)
Zuse paid for his projects out of his own pocket and constructed the first two machines in the living room of his parents’ apartment, with the help of a few close friends. His father was a post office clerk and didn’t have much money, but Zuse’s parents possessed a good deal of indulgent understanding; their son was obviously a rather original young man. While the world around him was going insane with Nazism, Zuse quietly submerged himself in his obsession, unaware of the work of like-minded engineers and scientists in the United States and Great Britain. He completed a prototype, later named the Z1, in 1938; a large jumble of moving plates, the machine was entirely mechanical and didn’t work very well, but it got him started in the right direction.
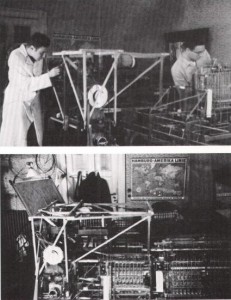
A la Jacquard and Babbage, the Z1 was controlled by punched tape. Instead of the usual gears and axles, the memory consisted of thin, slotted metal plates, the position of a pin in a slot – whether on the left or the right – representing a 0 or a 1. The memory contained more than a thousand plates, all cut by hand out of metal sheets, and stored about the same number of binary digits. It was the cleverest part of the Z1 and it operated satisfactorily, which was more than could be said of the arithmetic unit. Although the binary multiplication table is simplicity itself (0 x 0 = 0 and 1 x 1 = 1), Zuse never managed to get his mechanical arithmetic unit to carry and borrow efficiently or to link up well with the memory.
Confident of his design, Zuse set out to build a larger and more sophisticated calculator, the Z2. This machine was electromechanical. At the suggestion of Helmut Schreyer, an electrical engineer and Zuse’s most imaginative collaborator, Zuse replaced the balky mechanical parts of the Z1’s arithmetic unit with secondhand telephone relays. A relay is an on/off electromechanical switch. (Hollerith’s tabulators and sorters used relays.) Once widely used in telephone-switching circuits, it consists of an electromagnet (a coil of wire wrapped around a spool) that closes an electrical circuit when the power is applied. The use of relays not only enabled Zuse to construct an arithmetic unit that could carry and borrow reliably, but one that operated rather fast, since relays can turn on and off hundreds of times a minute. Zuse linked the new arithmetic unit to the mechanical memory and, 10 and behold, the whole thing worked, more or less.
If a calculator can be built out of relays, asked Schreyer, why not go one step further and make one out of vacuum tubes, which can switch on and off thousands of times a second? In the 1930s, tubes were used exclusively to amplify analog signals, like radio waves, and few people had thought of using them for digital applications – there weren’t very many. In 1919, two English scientists invented a circuit known as flip-flop, a pair of tubes that acted as a switch; in response to a suitable signal, one of the tubes flipped on while the other flopped off. (The tubes didn’t turn completely on or off but went into higher or lower states of energy; a cold tube can’t be switched on quickly and the act of turning tubes on and off tended to burn them out.) However, tubes were expensive and hard to come by in Germany in the late 1930s, and Zuse, who felt more comfortable with mechanical gadgets anyway, decided to stick with relays.
In 1941, Schreyer received a doctorate for a thesis on the use of tubes as digital switches. But the advent of World War II cut Germany off from the United States and England, and his dissertation ended up gathering dust on a library shelf and had no effect on the history of computers.
In 1939, Germany invaded Poland. Zuse, who was twenty-nine, was drafted. Did the mighty German war machine embrace the promise of Zuse’s calculator and, showering him with all the relays, tubes, and assistants he could use, proclaim: “Build us a computer with which Deutschland can bring the Allies to their knees?” Did Germany win the war? As Zuse recalled years later,
In 1939, due to the perfectly private state of my workshop and due to the lack of official sponsorship, I became a soldier at the beginning of the war. The manufacturer, who assisted me [a calculator maker who partially financed Zuse’s work], wrote a letter to my major requesting leave for me to complete my work on an important invention. He wrote that I was working on a machine useful for the calculations and designs in the aircraft industry. My major looked at this letter and said, “I don’t understand that. The German aircraft is the best in the world. I don’t see what to calculate further on.” Half a year later, I was freed from military service, not for work on computers but as an engineer in the aircraft industry.
Back at Henschel, Zuse finished the Z2 in his spare time. Meanwhile, Schreyer, who had not been drafted, pursued his own calculator plans. He managed to obtain about 150 tubes from the Telefunken Company and, financed by the Aerodynamics Research Institute, a major research organization, constructed a simple machine that could convert three-digit decimal numbers into binary numbers, and vice versa. In 1942, he submitted a proposal for an electronic calculator to the German Army Command; the computer would contain about 1,500 tubes and execute 10,000 operations a second. But Schreyer was turned down. Confident that the war would be over within two or three years, the army refused to fund any project that didn’t promise to contribute immediately to the war effort.

Zuse also asked the Aerodynamics Research Institute for help. The group wasn’t interested in a general-purpose calculator – almost no one in Germany was – but it had an urgent computational problem that Zuse might be able to help them with. The calculation of airplane wing flutter was tying up personnel and other badly needed resources and delaying aircraft production. Zuse said that he could design a special-purpose calculator to solve the necessary equations and asked for permission to build a prototype; actually, he intended to devise a general-purpose machine that could handle wing flutter equations along with other kinds of problems, and thereby prove that a general-purpose device was the way to go. Commissioned by the institute, he established a fifteen-man company and the Z3, the first operational general-purpose program-controlled calculator, was completed by December 1941.
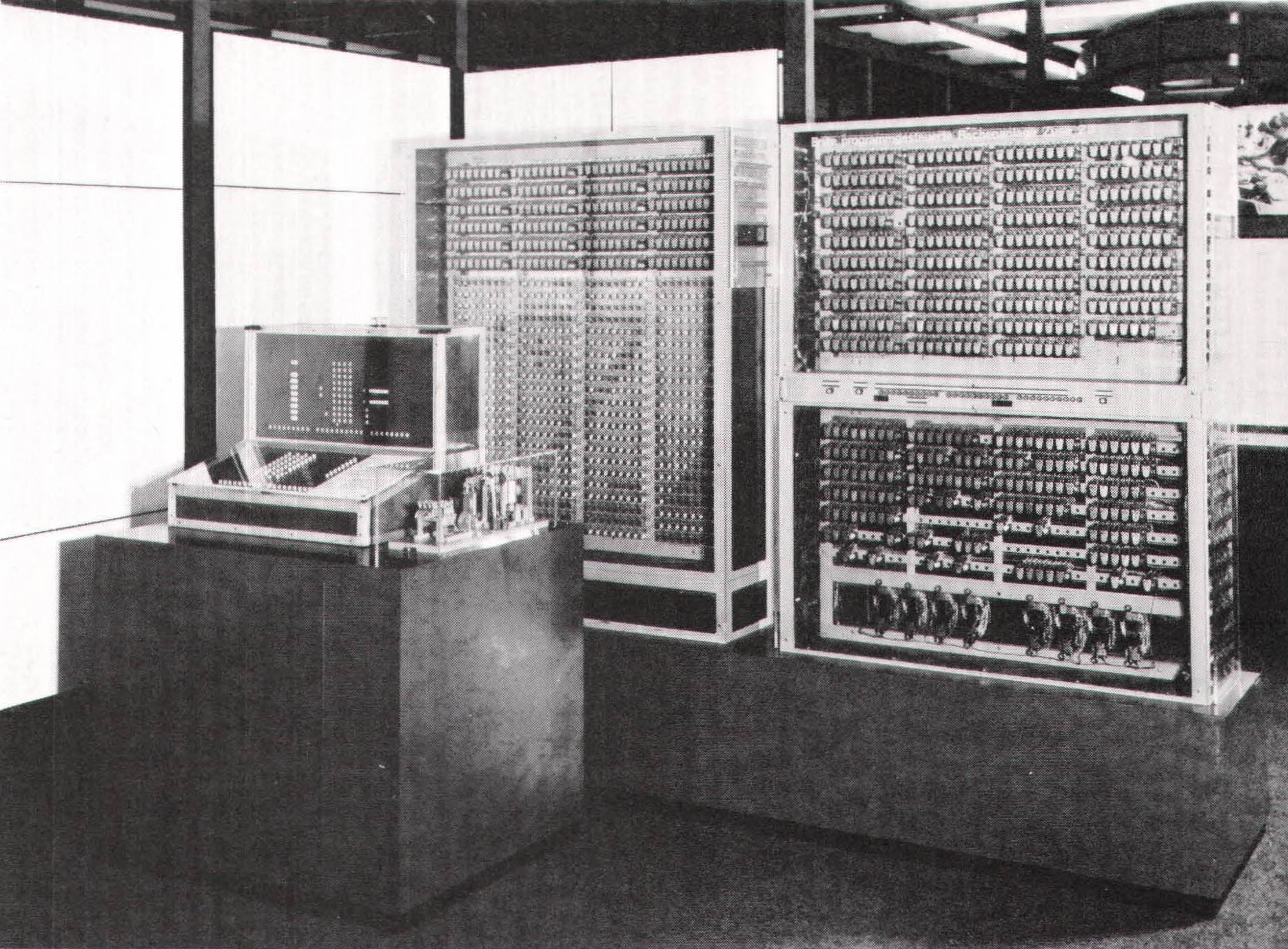
It was a small machine, consisting of a tape reader, an operator’s console, and two cabinets packed with 2,600 relays. It had a small memory, storing only sixty-four twenty-two-bit numbers, but it was rather fast, multiplying two words in only three to five seconds. In addition to the four basic operations of arithmetic, it could find square roots and carry out other complicated tasks automatically. However, it couldn’t execute conditional jumps. None of Zuse’s machines could; the idea never occurred to him. In a typical program, the initial values were entered into the memory by hand, an inconvenient method, and the ensuing operations were guided by punched tape. The cost of this great experiment in artificial computation? A mere $6,500 in materials.
By the way, the Z3 converted data into strings of twenty-two bits, which it stored and processed as separate units. In modern terminology, these twenty-two bit strings are called words. Word sizes vary from machine to machine; in many personal computers, for example, words contain sixteen bits.
Although the Z3 worked very well, the institute preferred special-purpose machines. So Zuse built two special-purpose calculators to analyze the wing flutter of flying bombs. Installed at Henschel, the machines were wired to carry out a fixed series of calculations on the bombs as they came off the assembly lines, indicating how each weapon’s wings should be adjusted. Despite their computational limitations, they proved to be quite efficient and the thirty women computers who had been employed to solve wing flutter equations with mechanical calculators were transferred to other jobs – a portent of the brave new world.
Henschel’s flying bomb shouldn’t be confused with the V-series of rocket bombs that Germany rained on Great Britain. Flying bombs were carried aloft by planes, released near their targets, and guided by radio signals from the aircraft. Fortunately, the bombs came along too late in the war to do much damage; beginning in August 1943, they were used against Allied ships in the Mediterranean and, two years later, against the Russians in the German retreat from Poland.